Within each atomic orbital subshell, every “filled” orbital consists of two entangled particles with opposing electromagnetic (e-m) directionality and interchanging identities to provide optimal directional balance. In other words, with each e-m interaction, the orbital particle with “electron” e-m directionality changes to “positron” e-m directionality, and its orbital partner with “positron” e-m directionality changes to “electron” e-m directionality. With the following e-m interaction, the each changes back to the opposite e-m directionality, the two entangled partners essentially interchanging identities with every e-m interaction.
Every orbital particle is not only entangled with its orbital partner, but also with a nuclear partner existing in a corresponding orbital within the nucleus. If the orbital particle is the only particle existing in its orbital, it will only be entangled with a nuclear partner, giving it less directional balance than if it was entangled with two particles, resulting in two entangled pairs of entangled orbital-nuclear partners with optimal directional balance. Since the single orbital particle possesses less directional balance, it is more likely to interact with another orbital particle of another atom to move toward greater directional balance.
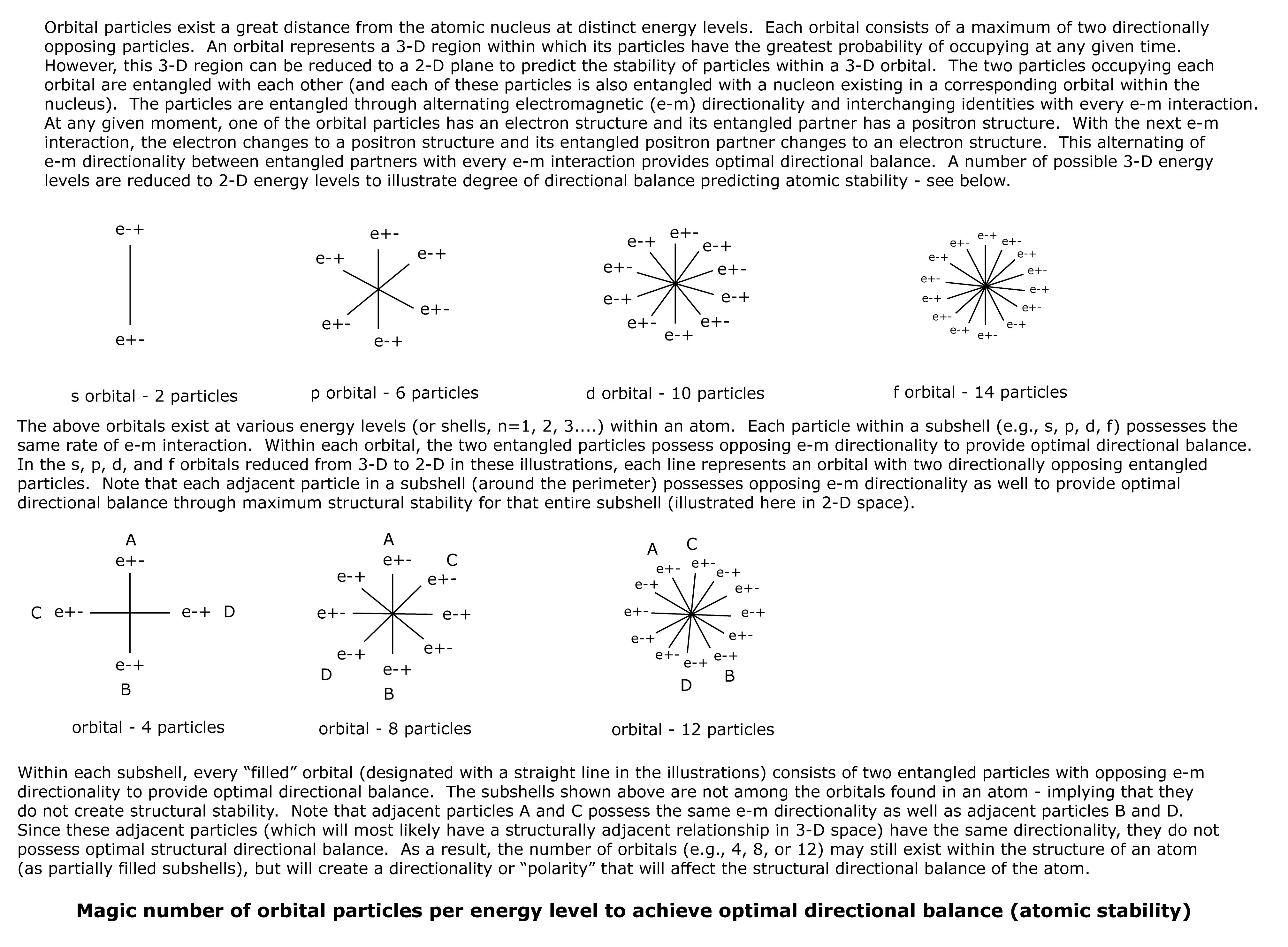
Depending upon size, atoms possess up to 5 or 6 energy levels, each consisting of sub-energy levels or subshells. The subshells are known as s, p, d, and f. The subshells consist of orbitals, and each orbital consists of a maximum of 2 orbital particles. The s subshell consists of 1 orbital, the p subshell consists of 3 orbitals, the d subshell consists of 5 orbitals, and the f subshell consists of 7 orbitals. This means that when each subshell is “filled” with the maximum number of orbital particles, the s subshell = 2 particles, the p subshell = 6 particles, the d subshell = 10 particles, and the f subshell = 14 particles.
Note that “unfilled” subshells may consist of an odd number of orbital particles, or of the following even number of orbital particles: 4, 8, or 12. This suggests that odd numbers of orbital particles or 4, 8, or 12 particles composing a subshell do not provide optimal directional balance and do not create optimal structural stability for the atom.
Each orbital consist of a region of 3-D space within which its particles have the highest probability of being found. However, 3-D space may be reduced to 2-D space to better illustrate the spatial relationship between adjacent orbital particles. In the illustration, each orbital of a subshell is represented by a straight line connecting the two particles in that orbital. When the subshell is “filled,” then the number of orbital particles in each subshell, s, p, d, and f is 2, 6, 10, and 14 respectively. When the arrangement of orbital particles for each subshell is shown in a 2-D space (see illustration), each adjacent particle possesses opposing e-m directionality with every e-m interaction. This suggests that the orbital particles in “filled” subshells provide optimal directional balance and optimal structural stability.
However, if a subshell possesses 4, 8, or 12 orbital particles, when shown in 2-D space (see illustration), there are two pairs of adjacent particles that possess the same e-m directionality with every e-m interaction. This suggests that subshells consisting of 4, 8, or 12 orbital particles do not possess optimal directional balance and do not provide optimal structural stability. Instead, they appear to produce a directionality or “polarity” that will affect the structural directional balance of the atom.
